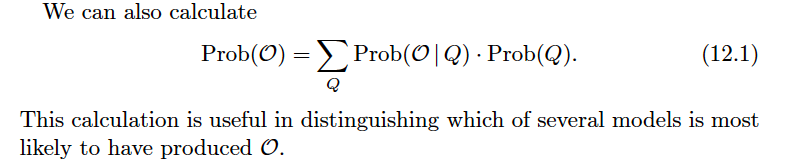0
$\begingroup$

I am reading "Statistical methods in bioinformatics"

I came across this formula: enter image description here

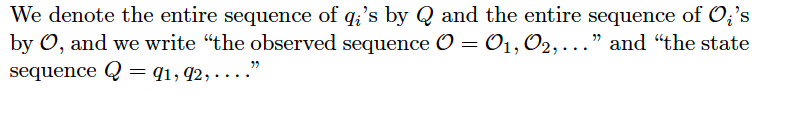
As explained in the book O are the observations: enter image description here

My question is why the author only specified $prob(O)$ for a sequence of length=1 ?, I assume he is only talking about one observation, but generally we have a sequence $O_1 ,O_2,O_3,...$.

I assume that $Prob(sequence)= P(O_1).P(O_2)...$ but I am not sure.

Improve this question
$\endgroup$
1
  • $\begingroup$ The capital non-indexed O seems to refer to the whole observed sequence (O = O1, O2, ...), so the probability P(O) is of observing the whole sequence of unspecified length. Or, am I missing something? $\endgroup$
    – Kamil S Jaron
    Commented Sep 2, 2020 at 12:43

1 Answer 1

Reset to default
2
$\begingroup$

The author writes in the quote that $O$ is to be taken for the whole set of positions $O_{i}$.

In the extracts that you provide there is no reason to believe that it is only for a sequence of length 1. The author writes "the observed sequence $O = O_1, O_2...$"

Thus as you say $Pr(sequence) = Pr(O) = Pr(O_1) * Pr(O_2)...$ according to my understanding of the quotes.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.